Argument map

An argument map or argument diagram is a visual representation of the structure of an argument. An argument map typically includes all the key components of the argument, traditionally called the conclusion and the premises, also called contention and reasons.^[1] Argument maps can also show co-premises, objections, counterarguments, rebuttals, and lemmas. There are different styles of argument map but they are often functionally equivalent and represent an argument's individual claims and the relationships between them.

Argument maps are commonly used in the context of teaching and applying critical thinking.^[2] The purpose of mapping is to uncover the logical structure of arguments, identify unstated assumptions, evaluate the support an argument offers for a conclusion, and aid understanding of debates. Argument maps are often designed to support deliberation of issues, ideas and arguments in wicked problems.^[3]

An argument map is not to be confused with a concept map or a mind map, two other kinds of node–link diagram which have different constraints on nodes and links.^[4]

Key features

A number of different kinds of argument maps have been proposed but the most common, which Chris Reed and Glenn Rowe called the standard diagram,^[5] consists of a tree structure with each of the reasons leading to the conclusion. There is no consensus as to whether the conclusion should be at the top of the tree with the reasons leading up to it or whether it should be at the bottom with the reasons leading down to it.^[5] Another variation diagrams an argument from left to right.^[6]

According to Douglas N. Walton and colleagues, an argument map has two basic components: "One component is a set of circled numbers arrayed as points. Each number represents a proposition (premise or conclusion) in the argument being diagrammed. The other component is a set of lines or arrows joining the points. Each line (arrow) represents an inference. The whole network of points and lines represents a kind of overview of the reasoning in the given argument..."^[7] With the introduction of software for producing argument maps, it has become common for argument maps to consist of boxes containing the actual propositions rather than numbers referencing those propositions.

There is disagreement on the terminology to be used when describing argument maps,^[8] but the standard diagram contains the following structures:

Dependent premises or co-premises, where at least one of the joined premises requires another premise before it can give support to the conclusion: An argument with this structure has been called a linked argument.^[9]

Statements 1 and 2 are dependent premises or co-premises.

Independent premises, where the premise can support the conclusion on its own: Although independent premises may jointly make the conclusion more convincing, this is to be distinguished from situations where a premise gives no support unless it is joined to another premise. Where several premises or groups of premises lead to a final conclusion the argument might be described as convergent. This is distinguished from a divergent argument where a single premise might be used to support two separate conclusions.^[10]

Statements 2, 3, 4 are independent premises.

Intermediate conclusions or sub-conclusions, where a claim is supported by another claim that is used in turn to support some further claim, i.e. the final conclusion or another intermediate conclusion: In the following diagram, statement 4 is an intermediate conclusion in that it is a conclusion in relation to statement 5 but is a premise in relation to the final conclusion, i.e. statement 1. An argument with this structure is sometimes called a complex argument. If there is a single chain of claims containing at least one intermediate conclusion, the argument is sometimes described as a serial argument or a chain argument.^[11]

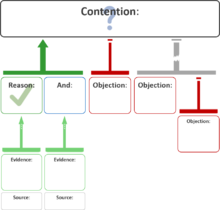
Statement 4 is an intermediate conclusion or sub-conclusion.

Each of these structures can be represented by the equivalent "box and line" approach to argument maps. In the following diagram, the contention is shown at the top, and the boxes linked to it represent supporting reasons, which comprise one or more premises. The green arrow indicates that the two reasons support the contention:

A box and line diagram

Argument maps can also represent counterarguments. In the following diagram, the two objections weaken the contention, while the reasons support the premise of the objection:

A sample argument using objections

Representing an argument as an argument map

Diagramming written text

A written text can be transformed into an argument map by following a sequence of steps. Monroe Beardsley's 1950 book Practical Logic recommended the following procedure:^[12]

Separate statements by brackets and number them.
Put circles around the logical indicators.
Supply, in parentheses, any logical indicators that are left out.
Set out the statements in a diagram in which arrows show the relationships between statements.

A diagram of the example from Beardsley's Practical Logic

Beardsley gave the first example of a text being analysed in this way:

Though ① [people who talk about the "social significance" of the arts don’t like to admit it], ② [music and painting are bound to suffer when they are turned into mere vehicles for propaganda]. For ③ [propaganda appeals to the crudest and most vulgar feelings]: (for) ④ [look at the academic monstrosities produced by the official Nazi painters]. What is more important, ⑤ [art must be an end in itself for the artist], because ⑥ [the artist can do the best work only in an atmosphere of complete freedom].

Beardsley said that the conclusion in this example is statement ②. Statement ④ needs to be rewritten as a declarative sentence, e.g. "Academic monstrosities [were] produced by the official Nazi painters." Statement ① points out that the conclusion isn't accepted by everyone, but statement ① is omitted from the diagram because it doesn't support the conclusion. Beardsley said that the logical relation between statement ③ and statement ④ is unclear, but he proposed to diagram statement ④ as supporting statement ③.

A box and line diagram of Beardsley's example, produced using Harrell's procedure

More recently, philosophy professor Maralee Harrell recommended the following procedure:^[13]

Identify all the claims being made by the author.
Rewrite them as independent statements, eliminating non-essential words.
Identify which statements are premises, sub-conclusions, and the main conclusion.
Provide missing, implied conclusions and implied premises. (This is optional depending on the purpose of the argument map.)
Put the statements into boxes and draw a line between any boxes that are linked.
Indicate support from premise(s) to (sub)conclusion with arrows.

Diagramming as thinking

Argument maps are useful not only for representing and analyzing existing writings, but also for thinking through issues as part of a problem-structuring process or writing process.^[14] The use of such argument analysis for thinking through issues has been called "reflective argumentation".^[15]

An argument map, unlike a decision tree, does not tell how to make a decision, but the process of choosing a coherent position (or reflective equilibrium) based on the structure of an argument map can be represented as a decision tree.^[16]

History

The philosophical origins and tradition of argument mapping

From Whately's Elements of Logic p467, 1852 edition

In the Elements of Logic, published in 1826 and issued in many subsequent editions,^[17] Archbishop Richard Whately gave probably the first form of an argument map, introducing it with the suggestion that "many students probably will find it a very clear and convenient mode of exhibiting the logical analysis of the course of argument, to draw it out in the form of a Tree, or Logical Division".

However, the technique did not become widely used, possibly because for complex arguments, it involved much writing and rewriting of the premises.

Wigmore evidence chart, from 1905

Legal philosopher and theorist John Henry Wigmore produced maps of legal arguments using numbered premises in the early 20th century,^[18] based in part on the ideas of 19th century philosopher Henry Sidgwick who used lines to indicate relations between terms.^[19]

Anglophone argument diagramming in the 20th century

Dealing with the failure of formal reduction of informal argumentation, English speaking argumentation theory developed diagrammatic approaches to informal reasoning over a period of fifty years.

Monroe Beardsley proposed a form of argument diagram in 1950.^[12] His method of marking up an argument and representing its components with linked numbers became a standard and is still widely used. He also introduced terminology that is still current describing convergent, divergent and serial arguments.

A Toulmin argument diagram, redrawn from his 1959 Uses of Argument

A generalised Toulmin diagram

Stephen Toulmin, in his groundbreaking and influential 1958 book The Uses of Argument,^[20] identified several elements to an argument which have been generalized. The Toulmin diagram is widely used in educational critical teaching.^[21]^[22] Whilst Toulmin eventually had a significant impact on the development of informal logic he had little initial impact and the Beardsley approach to diagramming arguments along with its later developments became the standard approach in this field. Toulmin introduced something that was missing from Beardsley's approach. In Beardsley, "arrows link reasons and conclusions (but) no support is given to the implication itself between them. There is no theory, in other words, of inference distinguished from logical deduction, the passage is always deemed not controversial and not subject to support and evaluation".^[23] Toulmin introduced the concept of warrant which "can be considered as representing the reasons behind the inference, the backing that authorizes the link".^[24]

Beardsley's approach was refined by Stephen N. Thomas, whose 1973 book Practical Reasoning In Natural Language^[25] introduced the term linked to describe arguments where the premises necessarily worked together to support the conclusion.^[26] However, the actual distinction between dependent and independent premises had been made prior to this.^[26] The introduction of the linked structure made it possible for argument maps to represent missing or "hidden" premises. In addition, Thomas suggested showing reasons both for and against a conclusion with the reasons against being represented by dotted arrows. Thomas introduced the term argument diagram and defined basic reasons as those that were not supported by any others in the argument and the final conclusion as that which was not used to support any further conclusion.

Scriven's argument diagram. The explicit premise 1 is conjoined with additional unstated premises a and b to imply 2.

Michael Scriven further developed the Beardsley-Thomas approach in his 1976 book Reasoning.^[27] Whereas Beardsley had said "At first, write out the statements...after a little practice, refer to the statements by number alone"^[28] Scriven advocated clarifying the meaning of the statements, listing them and then using a tree diagram with numbers to display the structure. Missing premises (unstated assumptions) were to be included and indicated with an alphabetical letter instead of a number to mark them off from the explicit statements. Scriven introduced counterarguments in his diagrams, which Toulmin had defined as rebuttal.^[29] This also enabled the diagramming of "balance of consideration" arguments.^[30]

In 1998 a series of large-scale argument maps released by Robert E. Horn stimulated widespread interest in argument mapping.^[31]

Development of computer-supported argument visualization

The argument map tree schema of Kialo with an example path through it: all Con-argument boxes and some Pros were emptied to illustrate an example path.^[32]

A partial argument tree with claims and impact votes for arguments illustrates one form of collective determination of argument weights that is based on equal-weight user voting.^[33] There is research into how to efficiently calculate the winning arguments or arguments' weights and the overall conclusions in digital argument map systems.^[34]

Human–computer interaction pioneer Douglas Engelbart, in a famous 1962 technical report on intelligence augmentation, envisioned in detail something like argument-mapping software as an integral part of future intelligence-augmenting computer interfaces:^[35]

You usually think of an argument as a serial sequence of steps of reason, beginning with known facts, assumptions, etc., and progressing toward a conclusion. Well, we do have to think through these steps serially, and we usually do list the steps serially when we write them out because that is pretty much the way our papers and books have to present them—they are pretty limiting in the symbol structuring they enable us to use. ... To help us get better comprehension of the structure of an argument, we can also call forth a schematic or graphical display. Once the antecedent-consequent links have been established, the computer can automatically construct such a display for us.
— Douglas Engelbart, "Augmenting human intellect: a conceptual framework" (1962)

In the middle to late 1980s, hypertext software applications that supported argument visualization were developed, including NoteCards and gIBIS; the latter generated an on-screen graphical hypertextual map of an issue-based information system, a model of argumentation developed by Werner Kunz and Horst Rittel in the 1970s.^[36] In the 1990s, Tim van Gelder and colleagues developed a series of software applications that permitted an argument map's premises to be fully stated and edited in the diagram, rather than in a legend.^[37] Van Gelder's first program, Reason!Able, was superseded by two subsequent programs, bCisive and Rationale.^[38]

Throughout the 1990s and 2000s, many other software applications were developed for argument visualization. By 2013, more than 60 such software systems existed.^[39] In a 2010 survey of computer-supported argumentation, Oliver Scheuer and colleagues noted that one of the differences between these software systems is whether collaboration is supported.^[40] In their survey, single-user argumentation systems included Convince Me, iLogos, LARGO, Athena, Araucaria, and Carneades; small group argumentation systems included Digalo, QuestMap, Compendium, Belvedere, and AcademicTalk; community argumentation systems included Debategraph and Collaboratorium.^[40] Free and open source structured argumentation systems include Argdown^[41] and Argüman.^[42]

As of 2020, the commercial website Kialo is the most widely adopted argumentation-based deliberation system with an argument-map interface.^[43] On Kialo, users can usually vote on the debate question to express their overall conclusion about the subject, with the average and a bar chart of these votes being included at the top of every debate. Moreover, users can rate the impact individual arguments at the top level had on their conclusion. In branches beneath the top level, users can likewise rank the impact any individual argument has on the claim above it. The rationale (i.e. the main causal arguments) for their vote on a thesis or an argument is not recorded if these reasons are missing in the claims beneath it or if these have not been rated by the same users.^[44] This system of transparent voting represents Kialo's algorithm of collective determination of argument weights and theses' veracities,^[45] which has a plurality component in that users of the site can also switch between the perspectives of specific users and several groups of users (e.g. supporters and opponents of a thesis) which for example enables identifying which arguments were considered as most impactful for these particular users.^[46] In the context of historical-political education, researcher Oliver Held identified at least five key components of historical judgment that can be implemented easily in Kialo: perspectivity, levels of relevance, interdependence, multi-causality and assessments.^[47]

Applications

Argument maps have been applied in many areas, but foremost in educational, academic and business settings, including design rationale.^[48] Argument maps are also used in forensic science,^[49] law, and artificial intelligence.^[50] It has also been proposed that argument mapping has a great potential to improve how we understand and execute democracy, in reference to the ongoing evolution of e-democracy.^[51]

Difficulties with the philosophical tradition

It has traditionally been hard to separate teaching critical thinking from the philosophical tradition of teaching logic and method, and most critical thinking textbooks have been written by philosophers. Informal logic textbooks are replete with philosophical examples, but it is unclear whether the approach in such textbooks transfers to non-philosophy students.^[21] There appears to be little statistical effect after such classes. Argument mapping, however, has a measurable effect according to many studies.^[52] For example, instruction in argument mapping has been shown to improve the critical thinking skills of business students.^[53]

Evidence that argument mapping improves critical thinking ability

There is empirical evidence that the skills developed in argument-mapping-based critical thinking courses substantially transfer to critical thinking done without argument maps. Alvarez's meta-analysis found that such critical thinking courses produced gains of around 0.70 SD, about twice as much as standard critical-thinking courses.^[54] The tests used in the reviewed studies were standard critical-thinking tests.

Limitations

When used with students in school, argument maps have limitations. They can "end up looking overly complex" and can increase cognitive load beyond what is optimal for learning the course content.^[55] Creating maps requires extensive coaching and feedback from an experienced argument mapper.^[55] Depending on the learning objectives, the time spent coaching students to create good maps may be better spent learning the course content instead of learning to diagram.^[55] When the goal is to prompt students to consider other perspectives and counterarguments, the goal may be more easily accomplished with other methods such as discussion, rubrics, and a simple argument framework or simple graphic organizer such as a vee diagram.^[55] To maximize the strengths of argument mapping and minimize its limitations in the classroom requires considering at what point in a learning progression the potential benefits of argument mapping would outweigh its potential disadvantages.^[55]

A 2022 security studies blog post said that "Kialo's simplicity does pose some weaknesses and limitations, and in general current [computer-supported argument visualization] systems cannot reliably automate analysis or synthesis of arguments in the same way that statistical packages can automate analysis of data".^[56]

Standards

Argument Interchange Format

The Argument Interchange Format, AIF, is an international effort to develop a representational mechanism for exchanging argument resources between research groups, tools, and domains using a semantically rich language.^[57] AIF-RDF is the extended ontology represented in the Resource Description Framework Schema (RDFS) semantic language. Though AIF is still something of a moving target, it is settling down.^[58]

Legal Knowledge Interchange Format

The Legal Knowledge Interchange Format (LKIF)^[59] was developed in the European ESTRELLA project^[60] and designed with the goal of becoming a standard for representing and interchanging policy, legislation and cases, including their justificatory arguments, in the legal domain. LKIF builds on and uses the Web Ontology Language (OWL) for representing concepts and includes a reusable basic ontology of legal concepts.

Argdown

Argdown is a Markdown-inspired lightweight markup language for complex argumentation.^[41] It is intended for exchanging arguments and argument reconstructions in a universally accessible and highly human-readable way. The Argdown syntax is accompanied by tools that facilitate coding and transform Argdown documents into argument maps.^[61]

Notes

^ Freeman 1991, pp. 49–90
^ For example: Davies 2012; Facione 2016, pp. 88–112; Fisher 2004; Kelley 2014, p. 73; Kunsch, Schnarr & van Tyle 2014; Walton 2013, p. 10; van Gelder 2015
^ For example: Culmsee & Awati 2013; Hoffmann & Borenstein 2013; Metcalfe & Sastrowardoyo 2013; Ricky Ohl, "Computer supported argument visualisation: modelling in consultative democracy around wicked problems", in Okada, Buckingham Shum & Sherborne 2014, pp. 361–380
^ For example: Davies 2010; Hunter 2008; Okada, Buckingham Shum & Sherborne 2014, pp. vii–x, 4
^ ^a ^b Reed & Rowe 2007, p. 64
^ For example: Walton 2013, pp. 18–20
^ Reed, Walton & Macagno 2007, p. 2
^ Freeman 1991, pp. 49–90; Reed & Rowe 2007
^ Harrell 2010, p. 19
^ Freeman 1991, pp. 91–110; Harrell 2010, p. 20
^ Beardsley 1950, pp. 18–19; Reed, Walton & Macagno 2007, pp. 3–8; Harrell 2010, pp. 19–21
^ ^a ^b Beardsley 1950
^ Harrell 2010, p. 28
^ This is related to the distinction between "knowledge telling" and "knowledge transforming" in composition studies: see, for example, Chryssafidou 2014, pp. 38–39, 413
^ For example: Hoffmann & Borenstein 2013; Hoffmann 2016; Hoffmann 2018
^ See section 4.2, "Argument maps as reasoning tools", in Brun & Betz 2016
^ Whately 1834 (first published 1826)
^ Wigmore 1913
^ Goodwin 2000
^ Toulmin 2003 (first published 1958)
^ ^a ^b Simon, Erduran & Osborne 2006
^ Böttcher & Meisert 2011; Macagno & Konstantinidou 2013
^ Reed, Walton & Macagno 2007, p. 8
^ Reed, Walton & Macagno 2007, p. 9
^ Thomas 1997 (first published 1973)
^ ^a ^b Snoeck Henkemans 2000, p. 453
^ Scriven 1976
^ Beardsley 1950, p. 21
^ Reed, Walton & Macagno 2007, pp. 10–11
^ van Eemeren et al. 1996, p. 175
^ Holmes 1999; Horn 1998; Robert E. Horn, "Infrastructure for navigating interdisciplinary debates: critical decisions for representing argumentation", in Kirschner, Buckingham Shum & Carr 2003, pp. 165–184
^ Bolton et al. 2020
^ Durmus, Ladhak & Cardie 2019
^ Young 2021
^ Engelbart 1962; For an account of Engelbart's place in the history of computer-supported argument visualization, see, e.g., Simon Buckingham Shum, "The roots of computer supported argument visualization", in Kirschner, Buckingham Shum & Carr 2003, pp. 3–24
^ Conklin & Begeman 1988, on gIBIS; Halasz 1988, on NoteCards; Kirschner, Buckingham Shum & Carr 2003, pp. 14–15, on the place of both in the history of computer-supported argument visualization
^ van Gelder 2007
^ Berg et al. 2009
^ Walton 2013, p. 11
^ ^a ^b Scheuer et al. 2010
^ ^a ^b See Voigt 2014. The Argdown website is argdown.org. Argdown is currently developed as an open source project: "christianvoigt/argdown: a simple syntax for complex argumentation". GitHub.com. Retrieved 2019-10-30.
^ Pührer 2017
^ De Liddo & Strube 2021
^ Carroll, Sun & Beck 2019
^ Durmus, Ladhak & Cardie 2019
^ Beck, Neupane & Carroll 2019
^ Held 2022
^ Kirschner, Buckingham Shum & Carr 2003; Okada, Buckingham Shum & Sherborne 2014
^ For example: Bex 2011
^ For example: Verheij 2005; Reed, Walton & Macagno 2007; Walton 2013
^ Hilbert 2009
^ Twardy 2004; Álvarez Ortiz 2007; Harrell 2008; Yanna Rider and Neil Thomason, "Cognitive and pedagogical benefits of argument mapping: LAMP guides the way to better thinking", in Okada, Buckingham Shum & Sherborne 2014, pp. 113–134; Dwyer 2011; Davies 2012
^ Carrington et al. 2011; Kunsch, Schnarr & van Tyle 2014
^ Álvarez Ortiz 2007, pp. 69–70 et seq
^ ^a ^b ^c ^d ^e Nussbaum 2012, pp. 125, 133
^ Durland 2022
^ See the AIF original draft description (2006) and the full AIF-RDF ontology specifications in RDFS format.
^ Bex et al. 2013
^ Boer, Winkels & Vitali 2008
^ "Estrella project website". estrellaproject.org. Archived from the original on 2016-02-12. Retrieved 2016-02-24.
^ Argdown tools include a web browser sandbox editor, an extension for Visual Studio Code, and a command-line tool; see "Getting started". argdown.org. Retrieved 2019-10-30.

References

Álvarez Ortiz, Claudia María (2007). Does philosophy improve critical thinking skills? (PDF) (M.A. thesis). Department of Philosophy, University of Melbourne. OCLC 271475715. Archived from the original (PDF) on 2012-07-08.
Beardsley, Monroe C. (1950). Practical logic. New York: Prentice-Hall. OCLC 4318971. A shorter version was published as Thinking Straight; the most recent edition is: Beardsley, Monroe C. (1975). Thinking straight: principles of reasoning for readers and writers (4th ed.). Englewood Cliffs, NJ: Prentice-Hall. ISBN 978-0139182358. OCLC 1168973.
Beck, Jordan; Neupane, Bikalpa; Carroll, John M. (July 2019). "Managing conflict in online debate communities: foregrounding moderators' beliefs and values on Kialo". First Monday. 24 (7). doi:10.5210/fm.v24i7.9585.
Berg, Timo ter; van Gelder, Tim; Patterson, Fiona; Teppema, Sytske (2009). Critical thinking: reasoning and communicating with Rationale. Amsterdam: Pearson Education Benelux. ISBN 978-9043018012. OCLC 301884530.
Bex, Floris J. (2011). Arguments, stories and criminal evidence: a formal hybrid theory. Law and philosophy library. Vol. 92. Dordrecht; New York: Springer. doi:10.1007/978-94-007-0140-3. ISBN 9789400701397. OCLC 663950184. S2CID 26791185.
Bex, Floris J.; Modgil, Sanjay; Prakken, Henry; Reed, Chris (2013). "On logical specifications of the Argument Interchange Format" (PDF). Journal of Logic and Computation. 23 (5): 951–989. doi:10.1093/logcom/exs033.
Boer, Alexander; Winkels, Radboud; Vitali, Fabio (2008). "MetaLex XML and the Legal Knowledge Interchange Format". In Casanovas, Pompeu; Sartor, Giovanni; Casellas, Núria; Rubino, Rossella (eds.). Computable models of the law: languages, dialogues, games, ontologies. Lecture Notes in Computer Science. Vol. 4884. Berlin; New York: Springer. pp. 21–41. doi:10.1007/978-3-540-85569-9_2. ISBN 9783540855682. OCLC 244765580. Retrieved 2016-02-24.
Bolton, Eric; Calderwood, Alex; Christensen, Niles; Kafrouni, Jerome; Drori, Iddo (1 December 2020). "High quality real-time structured debate generation". arXiv:2012.00209 [cs.CL].
Böttcher, Florian; Meisert, Anke (February 2011). "Argumentation in science education: a model-based framework". Science & Education. 20 (2): 103–140. Bibcode:2011Sc&Ed..20..103B. doi:10.1007/s11191-010-9304-5. S2CID 119954511.
Brun, Georg; Betz, Gregor (2016). "Analysing Practical Argumentation" (PDF). In Hansson, Sven Ove; Hirsch Hadorn, Gertrude (eds.). The argumentative turn in policy analysis: reasoning about uncertainty. Logic, argumentation & reasoning. Vol. 10. Cham; New York: Springer-Verlag. pp. 39–77. doi:10.1007/978-3-319-30549-3_3. ISBN 9783319305479. OCLC 950884495.
Carrington, Michal; Chen, Richard; Davies, Martin; Kaur, Jagjit; Neville, Benjamin (June 2011). "The effectiveness of a single intervention of computer-aided argument mapping in a marketing and a financial accounting subject". Higher Education Research & Development. 30 (3): 387–403. doi:10.1080/07294360.2011.559197. S2CID 144862766. Retrieved 2016-02-24.
Carroll, John M.; Sun, Na; Beck, Jordan (2019). "Creating dialectics to learn: infrastructures, practices, and challenges". In Díaz, Paloma; Ioannou, Andri; Bhagat, Kaushal Kumar; Spector, J. Michael (eds.). Learning in a digital world: perspective on interactive technologies for formal and informal education. Smart computing and intelligence. Singapore: Springer. pp. 37–58. doi:10.1007/978-981-13-8265-9_3. ISBN 9789811382642. S2CID 195785108.
Chryssafidou, Evangelia (May 2014). Argument diagramming and planning cognition in argumentative writing (Ph.D. thesis). Birmingham, UK: University of Birmingham. OCLC 890146780.
Conklin, E. Jeffrey; Begeman, Michael L. (October 1988). "gIBIS: a hypertext tool for exploratory policy discussion". ACM Transactions on Information Systems. 6 (4): 303–331. doi:10.1145/58566.59297. S2CID 2609461.
Culmsee, Paul; Awati, Kailash (2013) [2011]. "Chapter 7: Visualising reasoning, and Chapter 8: Argumentation-based rationale". The heretic's guide to best practices: the reality of managing complex problems in organisations. Bloomington, IN: iUniverse, Inc. pp. 153–211. ISBN 9781462058549. OCLC 767703320.
Davies, W. Martin (November 2010). "Concept mapping, mind mapping and argument mapping: what are the differences and do they matter?". Higher Education. 62 (3): 279–301. doi:10.1007/s10734-010-9387-6. S2CID 44953977.
Davies, W. Martin (Summer 2012). "Computer-aided argument mapping as a tool for teaching critical thinking". International Journal of Learning and Media. 4 (3–4): 79–84. doi:10.1162/IJLM_a_00106. S2CID 62373559.
De Liddo, Anna; Strube, Rosa (June 2021). "Understanding failures and potentials of argumentation tools for public deliberation". In Cech, Florian; Farnham, Shelly (eds.). C&T '21: Proceedings of the 10th International Conference On Communities & Technologies: Wicked Problems in the Age of Tech: June 21–25, 2021. New York: Association for Computing Machinery. pp. 75–88. doi:10.1145/3461564.3461584. ISBN 9781450390569. OCLC 1261323348. S2CID 235494842.
Durland, Harrison (30 November 2022). "Complexity demands adaptation: two proposals for facilitating better debate in international relations and conflict research". The Forum (blog) of the Georgetown Security Studies Review.
Durmus, Esin; Ladhak, Faisal; Cardie, Claire (2019). "The role of pragmatic and discourse context in determining argument impact". Proceedings of the 2019 Conference on Empirical Methods in Natural Language Processing and the 9th International Joint Conference on Natural Language Processing (EMNLP-IJCNLP). Hong Kong: Association for Computational Linguistics. pp. 5667–5677. arXiv:2004.03034. doi:10.18653/v1/D19-1568. S2CID 202768765.
Dwyer, Christopher Peter (2011). The evaluation of argument mapping as a learning tool (PDF) (Ph.D. thesis). School of Psychology, National University of Ireland, Galway. OCLC 812818648. Retrieved 2016-02-24.
van Eemeren, Frans H.; Grootendorst, Rob; Snoeck Henkemans, A. Francisca; Blair, J. Anthony; Johnson, Ralph H.; Krabbe, Erik C. W.; Plantin, Christian; Walton, Douglas N.; Willard, Charles A.; Woods, John (1996). Fundamentals of argumentation theory: a handbook of historical backgrounds and contemporary developments. Mahwah, NJ: Lawrence Erlbaum Associates. doi:10.4324/9780203811306. ISBN 978-0805818611. OCLC 33970847.
Engelbart, Douglas C. (1962). Augmenting human intellect: a conceptual framework. Menlo Park, CA: Stanford Research Institute. OCLC 8671016. Archived from the original on 2011-05-04. Retrieved 2018-04-09.
Facione, Peter A. (2016) [2011]. THINK critically (3rd ed.). Boston: Pearson. ISBN 978-0133909661. OCLC 893099404.
Fisher, Alec (2004) [1988]. The logic of real arguments (2nd ed.). Cambridge; New York: Cambridge University Press. doi:10.1017/CBO9780511818455. ISBN 978-0521654814. OCLC 54400059. Retrieved 2016-02-24.
Freeman, James B. (1991). Dialectics and the macrostructure of arguments: a theory of argument structure. Berlin; New York: Foris Publications. ISBN 978-3110133905. OCLC 24429943. Retrieved 2016-02-24.
van Gelder, Tim (2007). "The rationale for Rationale" (PDF). Law, Probability and Risk. 6 (1–4): 23–42. doi:10.1093/lpr/mgm032.
van Gelder, Tim (2015). "Using argument mapping to improve critical thinking skills". In Davies, Martin; Barnett, Ronald (eds.). The Palgrave handbook of critical thinking in higher education. New York: Palgrave Macmillan. pp. 183–192. doi:10.1057/9781137378057_12. ISBN 9781137378033. OCLC 894935460.
Goodwin, Jean (2000). "Wigmore's chart method". Informal Logic. 20 (3): 223–243. doi:10.22329/il.v20i3.2278.
Halasz, Frank G. (July 1988). "Reflections on NoteCards: seven issues for the next generation of hypermedia systems". Communications of the ACM. 31 (7): 836–852. CiteSeerX 10.1.1.124.2308. doi:10.1145/48511.48514.
Harrell, Maralee (December 2008). "No computer program required: even pencil-and-paper argument mapping improves critical-thinking skills" (PDF). Teaching Philosophy. 31 (4): 351–374. doi:10.5840/teachphil200831437. S2CID 7340407. Archived from the original (PDF) on 2013-12-29.
Harrell, Maralee (August 2010). "Creating argument diagrams". academia.edu.
Held, Oliver (May 2022). "Urteilsbildung digital mit Kialo – Wie die historische Urteilsbildung im Distanz- und Präsenzunterricht mit Kialo digital unterstützt werden kann (PRIMA-Modell)" [Digital judgment formation with Kialo: how historical judgment formation in distance and face-to-face teaching can be supported digitally with Kialo (PRIMA model)]. Geschichte Lernen (in German) (207): 54–57. Retrieved 2023-07-20.
Hilbert, Martin (April 2009). "The maturing concept of e-democracy: from e-voting and online consultations to democratic value out of jumbled online chatter" (PDF). Journal of Information Technology and Politics. 6 (2): 87–110. doi:10.1080/19331680802715242. S2CID 15790311.
Hoffmann, Michael H. G. (November 2016). "Reflective argumentation: a cognitive function of arguing". Argumentation. 30 (4): 365–397. doi:10.1007/s10503-015-9388-9. S2CID 146779158.
Hoffmann, Michael H. G. (March 2018). "Stimulating reflection and self-correcting reasoning through argument mapping: three approaches". Topoi. 37 (1): 185–199. doi:10.1007/s11245-016-9408-x. S2CID 123754143.
Hoffmann, Michael H. G.; Borenstein, Jason (February 2013). "Understanding ill-structured engineering ethics problems through a collaborative learning and argument visualization approach". Science and Engineering Ethics. 20 (1): 261–276. doi:10.1007/s11948-013-9430-y. PMID 23420467. S2CID 14247458.
Holmes, Bob (10 July 1999). "Beyond words". New Scientist. No. 2194. Archived from the original on 28 September 2008.
Horn, Robert E. (1998). Visual language: global communication for the 21st century. Bainbridge Island, WA: MacroVU, Inc. ISBN 978-1892637093. OCLC 41138655.
Hunter, Lawrie (2008). "Cmap linking phrase constraint for the structural narrowing of constructivist second language tasks" (PDF). In Cañas, Alberto J.; Reiska, Priit; Åhlberg, Mauri K.; Novak, Joseph Donald (eds.). CMC 2008: Third International Conference on Concept Mapping, Tallinn, Estonia and Helsinki, Finland, 22–25 September 2008: proceedings. Vol. 1. Tallinn: Tallinn University. pp. 146–151. ISBN 9789985585849.
Kelley, David (2014) [1988]. The art of reasoning: an introduction to logic and critical thinking (4th ed.). New York: W. W. Norton & Company. ISBN 978-0393930788. OCLC 849801096.
Kirschner, Paul Arthur; Buckingham Shum, Simon J; Carr, Chad S, eds. (2003). Visualizing argumentation: software tools for collaborative and educational sense-making. Computer supported cooperative work. New York: Springer. doi:10.1007/978-1-4471-0037-9. ISBN 978-1852336646. OCLC 50676911. S2CID 46267938. Retrieved 2016-02-24.
Kunsch, David W.; Schnarr, Karin; van Tyle, Russell (November 2014). "The use of argument mapping to enhance critical thinking skills in business education". Journal of Education for Business. 89 (8): 403–410. doi:10.1080/08832323.2014.925416. S2CID 38666976.
Macagno, Fabrizio; Konstantinidou, Aikaterini (August 2013). "What students' arguments can tell us: using argumentation schemes in science education". Argumentation. 27 (3): 225–243. doi:10.1007/s10503-012-9284-5. S2CID 189942834. SSRN 2185945.
Metcalfe, Mike; Sastrowardoyo, Saras (November 2013). "Complex project conceptualisation and argument mapping". International Journal of Project Management. 31 (8): 1129–1138. doi:10.1016/j.ijproman.2013.01.004.
Nussbaum, E. Michael (2012). "Argumentation and student-centered learning environments". In Jonassen, David H.; Land, Susan M. (eds.). Theoretical foundations of learning environments (2nd ed.). New York: Routledge. pp. 114–141. doi:10.4324/9780203813799. ISBN 9780415894210.
Okada, Alexandra; Buckingham Shum, Simon J; Sherborne, Tony, eds. (2014) [2008]. Knowledge cartography: software tools and mapping techniques. Advanced information and knowledge processing (2nd ed.). New York: Springer. doi:10.1007/978-1-4471-6470-8. ISBN 9781447164692. OCLC 890438015. S2CID 28008938. Retrieved 2016-02-24.
Pührer, Jörg (2017). "ArgueApply: a mobile app for argumentation". Logic Programming and Nonmonotonic Reasoning: 14th International Conference, LPNMR 2017, Espoo, Finland, July 3–6, 2017: proceedings. Lecture notes in computer science. Vol. 10377. Cham: Springer. pp. 250–262. doi:10.1007/978-3-319-61660-5_23. ISBN 978-3319616599.
Reed, Chris; Rowe, Glenn (2007). "A pluralist approach to argument diagramming" (PDF). Law, Probability and Risk. 6 (1–4): 59–85. doi:10.1093/lpr/mgm030. Retrieved 2016-02-24.
Reed, Chris; Walton, Douglas; Macagno, Fabrizio (March 2007). "Argument diagramming in logic, law and artificial intelligence". The Knowledge Engineering Review. 22 (1): 87. doi:10.1017/S0269888907001051. S2CID 26294789.
Scheuer, Oliver; Loll, Frank; Pinkwart, Niels; McLaren, Bruce M. (2010). "Computer-supported argumentation: a review of the state of the art" (PDF). International Journal of Computer-Supported Collaborative Learning. 5 (1): 43–102. CiteSeerX 10.1.1.322.2522. doi:10.1007/s11412-009-9080-x. S2CID 4473082.
Scriven, Michael (1976). Reasoning. New York: McGraw-Hill. ISBN 978-0070558823. OCLC 2800373.
Simon, Shirley; Erduran, Sibel; Osborne, Jonathan (2006). "Learning to teach argumentation: research and development in the science classroom" (PDF). International Journal of Science Education. 28 (2–3): 235–260. Bibcode:2006IJSEd..28..235S. doi:10.1080/09500690500336957. S2CID 145163500. Archived from the original (PDF) on 2015-09-19. Retrieved 2015-01-12.
Snoeck Henkemans, A. Francisca (November 2000). "State-of-the-art: the structure of argumentation". Argumentation. 14 (4): 447–473. doi:10.1023/A:1007800305762. S2CID 140364997.
Thomas, Stephen N. (1997) [1973]. Practical reasoning in natural language (4th ed.). Upper Saddle River, NJ: Prentice-Hall. ISBN 978-0136782698. OCLC 34745923.
Toulmin, Stephen E. (2003) [1958]. The uses of argument (Updated ed.). Cambridge; New York: Cambridge University Press. doi:10.1017/CBO9780511840005. ISBN 978-0521534833. OCLC 57253830. Retrieved 2016-02-24.
Twardy, Charles R. (June 2004). "Argument maps improve critical thinking" (PDF). Teaching Philosophy. 27 (2): 95–116. doi:10.5840/teachphil200427213.
Verheij, Bart (2005). Virtual arguments: on the design of argument assistants for lawyers and other arguers. Information technology & law series. Vol. 6. The Hague: T.M.C. Asser Press. ISBN 9789067041904. OCLC 59617214.
Voigt, Christian (2014). "Argdown and the stacked masonry layout: two user interfaces for non-expert users". In Parsons, Simon; Oren, Nir; Reed, Chris; Cerutti, Federico (eds.). Computational models of argument: proceedings of COMMA 2014. Frontiers in artificial intelligence and applications. Vol. 266. Amsterdam: IOS Press. pp. 483–484. doi:10.3233/978-1-61499-436-7-483. ISBN 9781614994367. OCLC 894508689.
Walton, Douglas N. (2013). Methods of argumentation. Cambridge; New York: Cambridge University Press. doi:10.1017/CBO9781139600187. ISBN 978-1107677333. OCLC 830523850. Retrieved 2016-02-24.
Whately, Richard (1834) [1826]. Elements of logic: comprising the substance of the article in the Encyclopædia metropolitana: with additions, &c (5th ed.). London: B. Fellowes. OCLC 1739330. Retrieved 2016-02-24.
Wigmore, John Henry (1913). The principles of judicial proof: as given by logic, psychology, and general experience, and illustrated in judicial trials. Boston: Little Brown. OCLC 1938596. Retrieved 2016-02-24.
Young, Anthony P. (September 2021). "Likes as argument strength for online debate" (PDF). argstrength2021.argumentationcompetition.org. Retrieved 2023-06-10.