Icositruncated dodecadodecahedron
(Redirected from Icosidodecatruncated icosidodecahedron)
| Icositruncated dodecadodecahedron | |
|---|---|
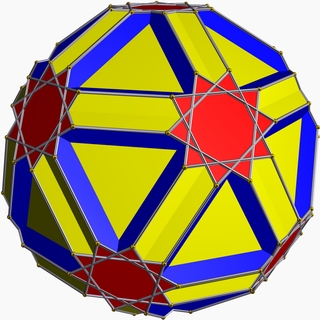
| |
| Type | Uniform star polyhedron |
| Elements | F = 44, E = 180 V = 120 (χ = −16) |
| Faces by sides | 20{6}+12{10}+12{10/3} |
| Coxeter diagram | |
| Wythoff symbol | 3 5 5/3 | |
| Symmetry group | Ih, [5,3], *532 |
| Index references | U45, C57, W84 |
| Dual polyhedron | Tridyakis icosahedron |
| Vertex figure |  6.10.10/3 |
| Bowers acronym | Idtid |
In geometry, the icositruncated dodecadodecahedron or icosidodecatruncated icosidodecahedron is a nonconvex uniform polyhedron, indexed as U45.

Convex hull
editIts convex hull is a nonuniform truncated icosidodecahedron.
| Truncated icosidodecahedron |
Convex hull |
Icositruncated dodecadodecahedron |
Cartesian coordinates
editCartesian coordinates for the vertices of an icositruncated dodecadodecahedron are all the even permutations of
where is the golden ratio.
Related polyhedra
editTridyakis icosahedron
edit| Tridyakis icosahedron | |
|---|---|
| Type | Star polyhedron |
| Face | |
| Elements | F = 120, E = 180 V = 44 (χ = −16) |
| Symmetry group | Ih, [5,3], *532 |
| Index references | DU45 |
| dual polyhedron | Icositruncated dodecadodecahedron |
The tridyakis icosahedron is the dual polyhedron of the icositruncated dodecadodecahedron. It has 44 vertices, 180 edges, and 120 scalene triangular faces.
See also
edit- Catalan solid Duals to convex uniform polyhedra
- Uniform polyhedra
- List of uniform polyhedra
References
edit- Wenninger, Magnus (1983), Dual Models, Cambridge University Press, ISBN 978-0-521-54325-5, MR 0730208 Photo on page 96, Dorman Luke construction and stellation pattern on page 97.
External links
edit