Wikipedia

User:Tomruen/Convex uniform tetracomb

< User:Tomruen

Regular and uniform honeycombs

edit

There are five fundamental affine Coxeter groups that generate regular and uniform tessellations in 4-space.[1]

# Coxeter group Coxeter-Dynkin diagram
1 A~4 [(3,3,3,3,3)] [3[5]]
2 B~4 [4,3,3,4]
3 C~4 [4,3,31,1] h[4,3,3,4]
4 D~4 [31,1,1,1] q[4,3,3,4]
5 F~4 [3,4,3,3] h[4,3,3,4]

There are three regular honeycomb of Euclidean 4-space:

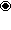
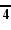
  1. tesseractic honeycomb, with symbols {4,3,3,4}, = . There are 19 uniform honeycombs in this family.
  2. 24-cell honeycomb, with symbols {3,4,3,3}, . There are 31 uniform honeycombs in this family.
  3. 16-cell honeycomb, with symbols {3,3,4,3},

Other families that generate uniform honeycombs:

  • There are 23 uniform honeycombs, 4 unique in the 16-cell honeycomb family. With symbols h{4,32,4} it is geometrically identical to the 16-cell honeycomb, =
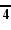
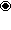
  • There are 7 uniform honeycombs from the A~4, family, all unique.
  • There are 9 uniform honeycombs in the D~4: [31,1,1,1] family, all repeated in other families, including the 16-cell honeycomb.

Non-Wythoffian uniform tessellations in 4-space also exist by elongation (inserting layers), and gyration (rotating layers) from these reflective forms.

The single-ringed tessellations are given below, indexed by Olshevsky's listing.

Duoprismatic forms

  • B~2xB~2: [4,4]x[4,4] = [4,3,3,4] = (Same as tesseractic honeycomb family)
  • B~2xH~2: [4,4]x[6,3]
  • H~2xH~2: [6,3]x[6,3]
  • A~2xB~2: [Δ]x[4,4] (Same forms as [6,3]x[4,4])
  • A~2xH~2: [Δ]x[6,3] (Same forms as [6,3]x[6,3])
  • A~2xA~2: [Δ]x[Δ] (Same forms as [6,3]x[6,3])

Prismatic forms

  • B~3xI~1: [4,3,4]x[∞]
  • D~3xI~1: [4,31,1]x[∞]
  • A~3xI~1:

Noncompact prismatic forms

  • A3xI~1: [3,3]x[∞] -
  • B3xI~1: [4,3]x[∞] -
  • H3xI~1: [5,3]x[∞] -
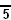
  • I~1xI~1xI2r: [∞] x [∞] x [r] = [4,4]x[r] - =

Non-Wythoffian forms

edit

The non-Wythoffian forms are built as stacked composites of these prismatic noncompact groups:

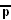
  • I2pxI~1xA1: [p]x[∞]x[ ] - (Prism column)
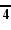
  • D~3xA1: [4,31,1]x[ ] (Prism slab)
  • A~3xA1: (Prism slab)
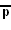
  • A~2xI2p: [Δ]x[p] (Prism slab)
  • B~2xI2p: [4,4]x[p] (Prism slab)
  • H~2xI2p: [6,3]x[p] (Prism slab)


B~4 [4,3,3,4] family

edit
# Coxeter-Dynkin
andSchläfli
symbols 		Name Facets by location: [4,3,3,4]
4 3 2 1 0

[4,3,3]
[4,3]×[ ]
[4]×[4]
[ ]×[3,4]
[3,3,4]
1
t0{4,3,3,4} 		Tesseractic honeycomb {4,3,3}
 - - - -
87
t1{4,3,3,4} 		Rectified tesseractic honeycomb t1{4,3,3}
 - - - {3,3,4}
88
t2{4,3,3,4} 		Birectified tesseractic honeycomb
(Same as 24-cell honeycomb {3,4,3,3}) 		t1{3,3,4}
 - - - t1{3,3,4}
89
t0,1{4,3,3,4} 		Truncated tesseractic honeycomb t0,1{4,3,3} - - - {3,3,4}
90
t0,2{4,3,3,4} 		Cantellated tesseractic honeycomb
(Small prismatotesseractic honeycomb) 		t0,2{4,3,3} - - {}x{3,4} t1{3,3,4}
91
t0,3{4,3,3,4} 		Runcinated tesseractic honeycomb
(Small diprismatotesseractic honeycomb)
92
t1,2{4,3,3,4} 		Bitruncated tesseractic honeycomb
93
t1,3{4,3,3,4} 		Bicantellated tesseractic honeycomb
(Same as Rectified 24-cell honeycomb t1{3,4,3,3})
[1]
t0,4{4,3,3,4} 		Stericated tesseractic honeycomb
(same as tesseractic honeycomb)
94
t0,1,2{4,3,3,4} 		Cantitruncated tesseractic honeycomb
(Great prismatotesseractic honeycomb)
95
t0,1,3{4,3,3,4} 		Runcitruncated tesseractic honeycomb
(Small tomocubic-diprismatotesseractic honeycomb)
96
t0,1,4{4,3,3,4} 		Steritruncated tesseractic honeycomb
(Tomotesseractic-diprismatotesseractic honeycomb)
97
t0,2,3{4,3,3,4} 		Runcicantellated tesseractic honeycomb
(Rhombitesseractic-diprismatotesseractic honeycomb)
98
t0,2,4{4,3,3,4} 		Stericantellated tesseractic honeycomb
(Small rhombitesseractic-prismatotesseractic honeycomb)
99
t1,2,3{4,3,3,4} 		Bicantitruncated tesseractic honeycomb
(Same as Truncated 24-cell honeycomb, t0,1{3,4,3,3} )
100
t0,1,2,3{4,3,3,4} 		Runcicantitruncated tesseractic honeycomb
(Great diprismatotesseractic honeycomb)
101
t0,1,2,4{4,3,3,4} 		Stericantitruncated tesseractic honeycomb
(Great rhombitesseractic-prismatotesseractic honeycomb)
102
t0,1,3,4{4,3,3,4} 		Steriruncitruncated tesseractic honeycomb
(Great tomocubic-diprismatotesseractic honeycomb)
103
t0,1,2,3,4{4,3,3,4} 		Omnitruncated tesseractic honeycomb

C~4 [31,1,3,4] family

edit

There are 23 honeycombs in this family,[2] all listed below.

# Coxeter-Dynkin
andSchläfli
symbols
File:CDel B5 nodes.png 		Name Facets by location: [31,1,3,4]
4 3 2 1 0

[3,3,4]
[31,1,1]
[3,3]×[ ]
[ ]×[3]×[ ]
[3,3,4]
104
{31,1,3,4} 		16-cell honeycomb {3,3,4}
 {31,1,1}
 - - -
105
t0,1{31,1,3,4} 		Truncated 16-cell honeycomb
(Same as truncated 16-cell honeycomb, t0,1{3,3,4,3})
106
t0,2{31,1,3,4} 		Cantellated 16-cell honeycomb
(Same as birectified 16-cell honeycomb)
107
t0,1,2{31,1,3,4} 		Cantitruncated 16-cell honeycomb
(Same as bitruncated 16-cell honeycomb)
108
t0,3{31,1,3,4} 		Runcinated 16-cell honeycomb
(Small diprismatodemitesseractive honeycomb)
109
t0,1,3{31,1,3,4} 		Runcitruncated 16-cell honeycomb
(Small prismato16-cell honeycomb)
110
t0,2,3{31,1,3,4} 		Runcicantellated 16-cell honeycomb
(Great prismato16-cell honeycomb)
111
t0,1,2,3{31,1,3,4} 		Runcicantitruncated 16-cell honeycomb
(Great diprismato16-cell honeycomb)
[88]
t1{31,1,3,4} 		Rectified 16-cell honeycomb
(Same as 24-cell honeycomb, {3,4,3,3})
(Also birectified tesseractic honeycomb) 		t1{3,3,4}
 t1{31,1,1}

 - - t1{3,3,4}
[87]
t2{31,1,3,4} 		Same as rectified tesseractic honeycomb t1{4,3,3}
 {31,1,1}
 - - t1{4,3,3}
[1]
t3{31,1,3,4} 		Same as tesseractic honeycomb {4,3,3}
 - - - {4,3,3}
[87]
t0,4{31,1,3,4} 		(Same as Rectified tesseractive honeycomb)
[92]
t1,2{31,1,3,4} 		(Same as bitruncated tesseractic honeycomb)
[90]
t1,3{31,1,3,4} 		(Same as cantellated tesseractic honeycomb)
[89]
t2,3{31,1,3,4} 		(Same as truncated tesseractic honeycomb)
[92]
t0,1,4{31,1,3,4} 		(Same as bitruncated tesseractic honeycomb)
[93]
t0,2,4{31,1,3,4} 		(Same as rectified 24-cell honeycomb)
(Also bicantellated tesseractic honeycomb)
[91]
t0,3,4{31,1,3,4} 		(Same as runcinated tesseractic honeycomb)
[94]
t1,2,3{31,1,3,4} 		(Same as cantitruncated tesseractic honeycomb)
[99]
t0,1,2,4{31,1,3,4} 		(Same as bicantitruncated tesseractic honeycomb)
[97]
t0,1,3,4{31,1,3,4} 		(Same as runcicantellated tesseractic honeycomb)
[95]
t0,2,3,4{31,1,3,4} 		(Same as runcitruncated tesseractic honeycomb)
[100]
t0,1,2,3,4{31,1,3,4} 		(Same as runcicantitruncated tesseractic honeycomb)

F~4 [3,4,3,3] family

edit

There are 32 honeycombs in this family, 31 reflective forms and one snub.[3] They are named as truncated forms from the regular 16-cell honeycomb and 24-cell honeycomb. These 31 forms are listed by the regular generators in two groups of 19, with 7 shared between.

From the regular 24-cell honeycomb, 19 forms are:

# Coxeter-Dynkin
andSchläfli
symbols 		Name Facets by location: [3,4,3,3]
4 3 2 1 0

[3,4,3]
[3,4]×[ ]
[3]×[3]
[ ]×[3,3]
[4,3,3]
104
{3,4,3,3} 		24-cell honeycomb {3,4,3} - - - -
[93]
t1{3,4,3,3} 		Rectified 24-cell honeycomb t1{3,4,3} - - - {4,3,3}
106
t2{3,4,3,3} 		Birectified 24-cell honeycomb t1{3,4,3} - - - t1{4,3,3}
106
t2{3,4,3,3} 		Birectified 24-cell honeycomb
105
t0,1{3,4,3,3} 		Truncated 24-cell honeycomb
107
t1,2{3,4,3,3} 		Bitruncated 24-cell honeycomb
?
t0,2{3,4,3,3} 		Cantellated 24-cell honeycomb
116
t1,3{3,4,3,3} 		Bicantellated 24-cell honeycomb
122
t0,3{3,4,3,3} 		Runcinated 24-cell honeycomb
121
t0,4{3,4,3,3} 		Stericated 24-cell honeycomb
[99]
t0,1,2{3,4,3,3} 		Cantitruncated 24-cell honeycomb
119
t1,2,3{3,4,3,3} 		Bicantitruncated 24-cell honeycomb
128
t0,1,3{3,4,3,3} 		Runcitruncated 24-cell honeycomb
125
t0,2,3{3,4,3,3} 		Runcicantellated 24-cell honeycomb
127
t0,1,4{3,4,3,3} 		Steritruncated 24-cell honeycomb
124
t0,2,4{3,4,3,3} 		Stericantellated 24-cell honeycomb
131
t0,1,2,3{3,4,3,3} 		Runcicantitruncated 24-cell honeycomb
130
t0,1,2,4{3,4,3,3} 		Stericantitruncated 24-cell honeycomb
129
t0,1,3,4{3,4,3,3} 		Steriruncitruncated 24-cell honeycomb
132
t0,1,2,3,4{3,4,3,3} 		Omnitruncated 24-cell honeycomb
[133]
t0,1,2{3,3,4,3} 		Snub 24-cell honeycomb

From the regular 16-cell honeycomb, 19 forms are:

# Coxeter-Dynkin
andSchläfli
symbols 		Name Facets by location: [3,3,4,3]
4 3 2 1 0

[3,3,4]
[3,3]×[ ]
[3]×[3]
[ ]×[4,3]
[3,4,3]
88
t0{3,3,4,3} 		16-cell honeycomb
[104]
t1{3,3,4,3} 		Rectified 16-cell honeycomb t1{3,3,4} - - - {3,4,3}
[106]
t2{3,3,4,3} 		Birectified 16-cell honeycomb
[99]
t0,1{3,3,4,3} 		Truncated 16-cell honeycomb
113
t1,2{3,3,4,3} 		Bitruncated 16-cell honeycomb
112
t0,2{3,3,4,3} 		Cantellated 16-cell honeycomb
[116]
t1,3{3,3,4,3} 		Bicantellated 16-cell honeycomb
115
t0,3{3,3,4,3} 		Runcinated 16-cell honeycomb
[121]
t0,4{3,3,4,3} 		Stericated 16-cell honeycomb
114
t0,1,2{3,3,4,3} 		Cantitruncated 16-cell honeycomb
[119]
t1,2,3{3,3,4,3} 		Bicantitruncated 16-cell honeycomb
117
t0,1,3{3,3,4,3} 		Runcitruncated 16-cell honeycomb
118
t0,2,3{3,3,4,3} 		Runcicantellated 16-cell honeycomb
123
t0,1,4{3,3,4,3} 		Steritruncated 16-cell honeycomb
[124]
t0,2,4{3,3,4,3} 		Stericantellated 16-cell honeycomb
120
t0,1,2,3{3,3,4,3} 		Runcicantitruncated 16-cell honeycomb
126
t0,1,2,4{3,3,4,3} 		Stericantitruncated 16-cell honeycomb
[129]
t0,1,3,4{3,3,4,3} 		Steriruncitruncated 16-cell honeycomb
[132]
t0,1,2,3,4{3,3,4,3} 		Omnitruncated 16-cell honeycomb
[133]
t0,1{3,4,3,3} 		Snub 24-cell honeycomb

A~4 [3[5]] family

edit

There are 7 honeycombs in this family,[4] all unique to this family, all given below.

# Coxeter-Dynkin
andSchläfli
symbols 		Name Facets by location
4 3 2 1 0

[3,3,3]
[ ]x[3,3]
[3]x[3]
[3,3]x[ ]
[3,3,3]
134
{3[5]} 		5-cell honeycomb
135
t0,1{3[5]} 		Truncated 5-cell honeycomb
136
t0,2{3[5]} 		Cantellated 5-cell honeycomb
137
t0,1,2{3[5]} 		Cantitruncated 5-cell honeycomb
138
t0,1,3{3[5]} 		Runcitruncated 5-cell honeycomb
139
t0,1,2,3{3[5]} 		Runcicantitruncated 5-cell honeycomb
140
t0,1,2,3,4{3[5]} 		Omnitruncated 5-cell honeycomb

D~4 [31,1,1,1] family

edit

There are 9 honeycombs in this family,[5] all repeated, with all 9 forms given below.

# Coxeter-Dynkin
andSchläfli
symbols 		Name Facets by location: [3,4,3,3]
4 3 2 1 0
[?]
{31,1,1,1} 		-
[104]
t4{31,1,1,1} 		Same as 24-cell honeycomb - t1{31,1,1}

 t1{31,1,1}

 t1{31,1,1}

 t1{31,1,1}

[?]
t0,4{31,1,1,1} 		-
[?]
t0,1{31,1,1,1} 		-
[?]
t0,1,4{31,1,1,1} 		-
[?]
t0,1,2{31,1,1,1} 		-
[?]
t0,1,4{31,1,1,1} 		-
[?]
t0,1,2,3{31,1,1,1} 		{}x{}x{}x{}
t0,2,3{31,1,1}

 t0,2,3{31,1,1}

 t0,2,3{31,1,1}

 t0,2,3{31,1,1}

[?]
t0,1,2,3,4{31,1,1,1} 		{}x{}x{}x{}
 t0,1,2,3{31,1,1}

 t0,1,2,3{31,1,1}

 t0,1,2,3{31,1,1}

 t0,1,2,3{31,1,1}

Duoprismatic forms

edit

Coxeter groups:

  • B~2xB~2: [4,4]x[4,4]
  • B~2xH~2: [4,4]x[6,3]
  • H~2xH~2: [6,3]x[6,3]

[4,4]×[4,4]

edit

There are 15 reflective combinatoric forms, but only 3 unique ones.

# Coxeter-Dynkin
andSchläfli
symbols 		Name
1
[1]
6
[1]
[6]
[1]
[6]
[1]
[6]
63
[6]
[63]
[1]
[6]
[63]
10
[10]
67
[10]
[67]

[4,4]x[6,3]

edit

There are 35 reflective combinatoric forms.

# Coxeter-Dynkin Name

[6,3]x[6,3]

edit

There are 28 reflective combinatoric forms.

# Coxeter-Dynkin diagram Name

References

edit
  1. ^ George Olshevsky (2006), Uniform Panoploid Tetracombs, manuscript. Complete list of 11 convex uniform tilings, 28 convex uniform honeycombs, and 143 convex uniform tetracombs.
  2. ^ Olshevsky section V
  3. ^ Olshevsky section VI
  4. ^ Olshevsky section VII
  5. ^ Olshevsky section VII
edit
Retrieved from "https://en.wikipedia.org/w/index.php?title=User:Tomruen/Convex_uniform_tetracomb&oldid=571197257"